I was musing as I was preparing for next week's Intensive at BGI that I have 21 students in my class, an uncomfortable size. That's because it lies between a smaller size where good conversations naturally occur, and a larger size where you can take full advantage of different activities that work well for larger groups. I talk about this a bit in my Group Threshold and Dunbar Number posts, where I call the group threshold size of between 10 and 24 people the “Judas Number” nadir, or low point.

In my first article in this series I talked about community numbers: how the sizes of groups ultimately affect their success (or failure). However what I discussed only offers up the most rudimentary explanation of the dynamics, and that is because typically not all of the members of a group are equally involved. In order to better define who constitutes the tightly-knit "participant community" upon which the group thresholds act, we have to study power laws which let us measure the intensity of individuals' involvement in a group.

We often think of communities as organic creatures, which come into existence and grow on their own. However, the truth is they are fragile blossoms. Although many communities surely germinate and bloom on their own, purposefully creating communities can take a tremendous amount of hard work, and one factor their success ultimately depends upon is their numbers. If a community is too small you'll often have insufficient critical mass to sustain it.

Last May I did an abbreviated version of my Dunbar Number talk at MeshForum 2006. A MP3 podcast of that talk is now available at IT Conversations  or can be downloaded here (10mb). If you'd like to follow along, here is a pdf copy of my presentation sides (10mb). Biggest addition to what I've written about before is some discussion about different kinds of social software and what what size groups they seem to be appropriate for.

I will be speaking next Tuesday (July 11th) at the monthly meeting of BayCHI, the San Francisco Bay Area Chapter of ACM SIGCHI (Computer Human Interface Special Interest Group), along with Michael H. Goldhaber. The synopsis of my topic is: The Dunbar Number, Unstructured Trust, and Why Groups Don't Scale We are relying increasingly on internet-mediated social software tools for our day-to-day interaction with other people. To design this type of software, we must better understand the psychology and social dynamics of individuals in groups.

There is some more excellent research this week by Nick Yee and Nicolas Ducheneaut in the PlayOn blog. Again, their research provides good insight into social group dynamics as they appear in online games. I last mentioned their research on guild sizes in my blog post Dunbar & World of Warcraft where I compare the distribution of guild sizes in Ultima Online vs PlayOn's results from World of Warcraft. However, both distribution tables suffer from a variety of biases due to the nature of the different game designs, many of which are discussed in the commments in the post.

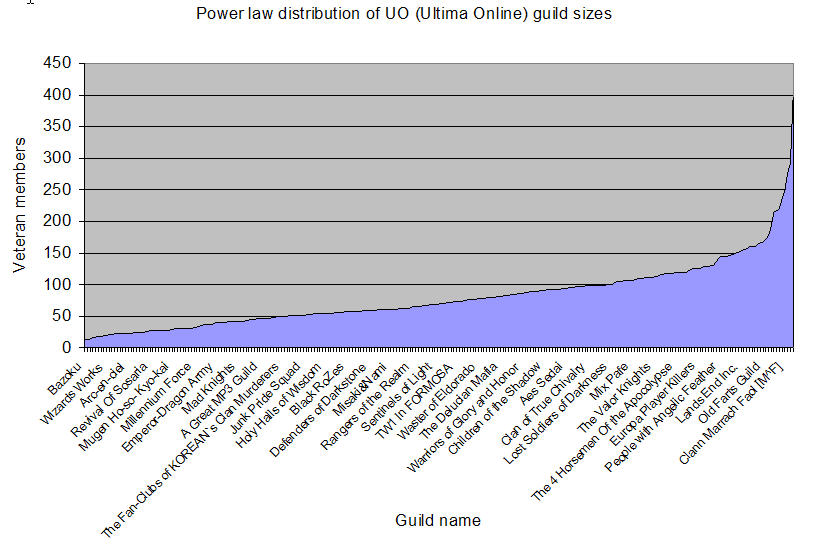In my initial blog entry on the Dunbar Number I presented some statistics on group sizes based on the online game Ultimata Online. In it you could clearly see the power-law (pareto) curve, with diminishing returns at around 150, with most groups being 60 in size: More recently, Nick Yee and other researchers at the PlayOn Blog have been researching the behaviors of players in the popular World of Warcraft online game.

In yesterday's All Things Considered, NPR commentator Jake Halpern questions why you feel like you have a relationship with the characters in your favorite TV shows. His answer: "That's because you do." This idea is interesting not just to help us understand how we relate to imaginary characters, but also to correlate with our own understanding of Dunbar Number relationship limits and how they might relate to this concept. In his commentary, Bostonian Jake Halpern considers two academic ideas, "belongingness theory" and "para-social relationships", and how they affect the relationships we develop with television characters.

In my post about the Dunbar Number I offered some evidence on the levels of satisfaction of various group sizes based on some empirical data from online games. There I was able to show that even though the Dunbar Number might predict a mean group size of 150 for humans, that in fact for non-survival oriented groups the mean was significantly less, probably between 60 to 90. I also offered a second hypothesis, that there is a dip in satisfaction level of groups at around the size of 15.

As someone who now has over 171 professional "connections" in my LinkedIn Profile, 198 "friends" on Orkut, many more non-intersecting friends and acquaintances on   Tribe.Net, LiveJournal, and other social networking services, as well as a plethora of correspondents that I only interact with via email, I am trying reconcile a mismatch between my connections and my own Dunbar Number. How do I maintain meaningful relationships with over 300 people?